7 Tips for Grade 9 Algebra

The transition from Grade 8 math to Grade 9 is quite significant, as students begin to learn the foundation for the rest of their math courses in high school. Students in Grade 9 will be introduced to Algebra and Functions, two new and very important concepts. If you are in Grade 9 and want to master Algebra, you’ve come to the right place. Here are some tips and tricks for Grade 9 Algebra.

1. Do practice problems as often as possible. 

• The best way to ace any Algebra problem that comes your way is to practice.
• The more practice problems you do, the more comfortable you’ll become at solving equations. 
• Start with more simple problems and work your way up to more challenging ones. You need to be able to solve and understand simpler equations before tackling complex problems. 

2. It’s good to make mistakes.

• While you’re doing practice problems, go over your mistakes. Making mistakes is the best way to learn. 
• Make sure you address your mistakes early, as this will help you understand how to tackle tricky problems in the future. 
• If you don’t quite understand your mistake, ask your teacher or a tutor for some help! Properly understanding your error will help you avoid making the same mistake again on another Algebra problem.

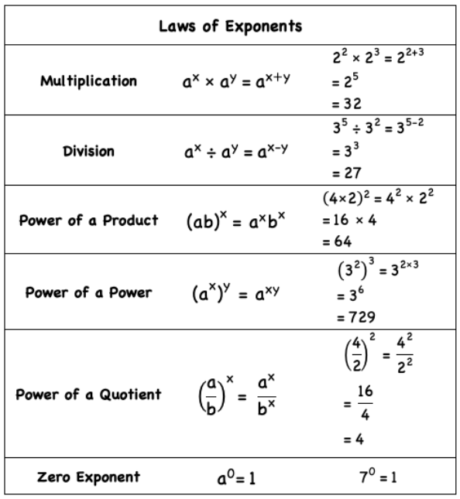
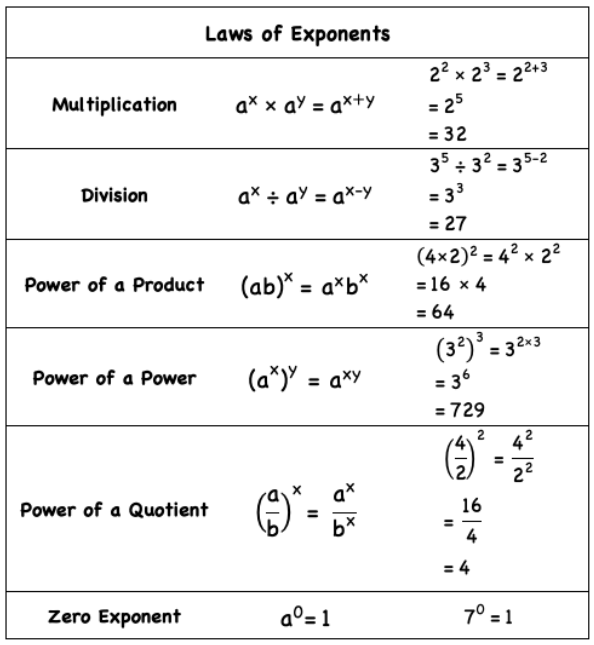
3. Familiarize yourself with the laws of exponents.

• While you may have seen exponents in your previous math classes, in Grade 9, you will be learning how to solve algebraic expressions that involve exponents.
• This means you will come across addition, subtraction, multiplication, and division problems that add exponents to the mix. 
• Exponents have crucial rules to follow in order to solve these problems without mistakes. They are called the laws of exponents.
• Practice solving algebraic expressions with exponents as often as possible. It’s better that you properly understand the laws of exponents rather than memorizing them.
• If you follow the laws of exponents and apply them to your practice problems, you will easily be able to solve any algebraic expression.
  

4. Practice solving polynomial expressions.

• What is a polynomial? A polynomial is an expression that includes variables and coefficients. 
• For instance, 3x2 – 5y + 2 is a polynomial expression. The 3 and 5 are coefficients, and the x and y are variables. 
• In Grade 9, students will learn how to solve polynomial expressions to find the value of the variable.
• Let’s try it out with the following polynomial: y = 5x + 15. To solve this problem, we need to isolate x on one side of the equation. 
• To begin, always make sure the expression is equal to zero. Now, we have 5x + 15 = 0. 
• Our next step involves moving the constant over to the zero side, which gives us
  5x = –15. 
• Our final step requires us to separate the 5 from the x variable. To remove the 5, we need to divide it on both sides of the equation. This gives us x = –15 ÷ 5. Therefore, our final answer is x = –3. 
• Remember: whatever you do to one side of the equation, you must do to the other side.

5. Get to know the order of operations.

• Grade 9 Math involves problems with several operations in the same expression. This means a math expression that includes exponents, brackets, additions, multiplications, and so on.
• It’s essential to understand the rules of BEDMAS if you’re tackling an algebraic expression. “BEDMAS” stands for Brackets, Exponents, Division, Multiplication, Addition, and Subtraction. 
• When coming across a math problem with several operations, you must start with brackets and move on to the next operation.  
• If you follow the order of BEDMAS, you will be able to solve any math problem that comes your way.

6 . Practice working with positive and negative numbers.

• Algebra often involves solving problems that have positive and negative numbers. These can often be confusing for students, but with enough practice, you’ll be an expert in no time!
• If you’re a visual learner, try using a number line so you can visualize the positive and negative numbers. 
• There are a few rules to follow when it comes to adding and subtracting positive and negative numbers. 
• Adding a negative number to a positive number is the same as subtracting a positive number from another positive number. Here’s an example: (+8) + (–3)= (+5) is the same as (+8) – (+3) = (+5).
• Subtracting a negative number to a positive number is the same as adding a positive number to another positive number. Here’s an example: (+3) – (–7) = (+10) is the same as (+3) + (+7) = (+10). 
• If the signs in the equation are the same (+ and +, or – and –), it becomes a positive sign.
• If the signs in the equation are different (+ and –, or – and +), it becomes a negative sign.

7. Ask for help.

• If you aren’t sure about how to solve a practice problem, ask your teacher or a friend for help. 
• The best time to ask questions is when you’re practicing math problems, so you’re well prepared for quizzes and exams.
• Compare your answers with your friend’s answers. Sometimes the best way to learn is by teaching others!
• Make sure you understand your mistakes so you don’t repeat them in the future. Many students make small mistakes that can be avoided with extra practice and understanding.

If you follow the above tips and practice math problems as often as possible, you will have everything you need to succeed in Grade 9 Algebra. Remember that Grade 9 Math is challenging and requires a lot of hard work to succeed. Be patient and confident in your abilities! At Success Tutorial School, we offer several programs to assist students who are taking or are planning on taking Grade 9 Math. Contact us to learn more about how we can help you or your child achieve great success in Grade 9 Math.